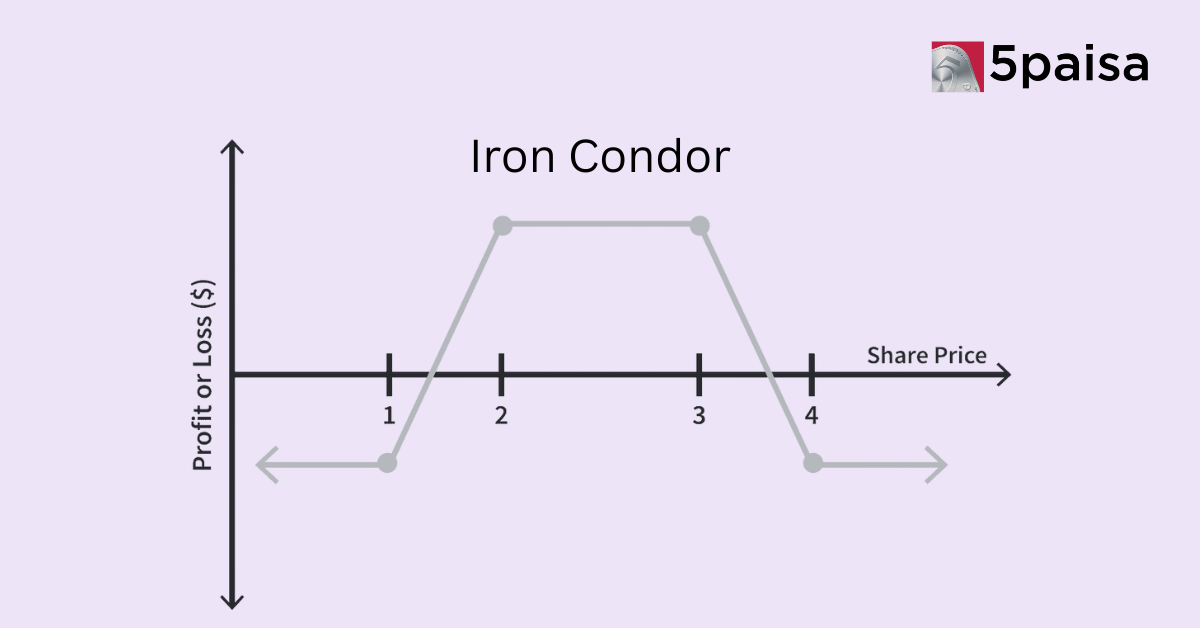
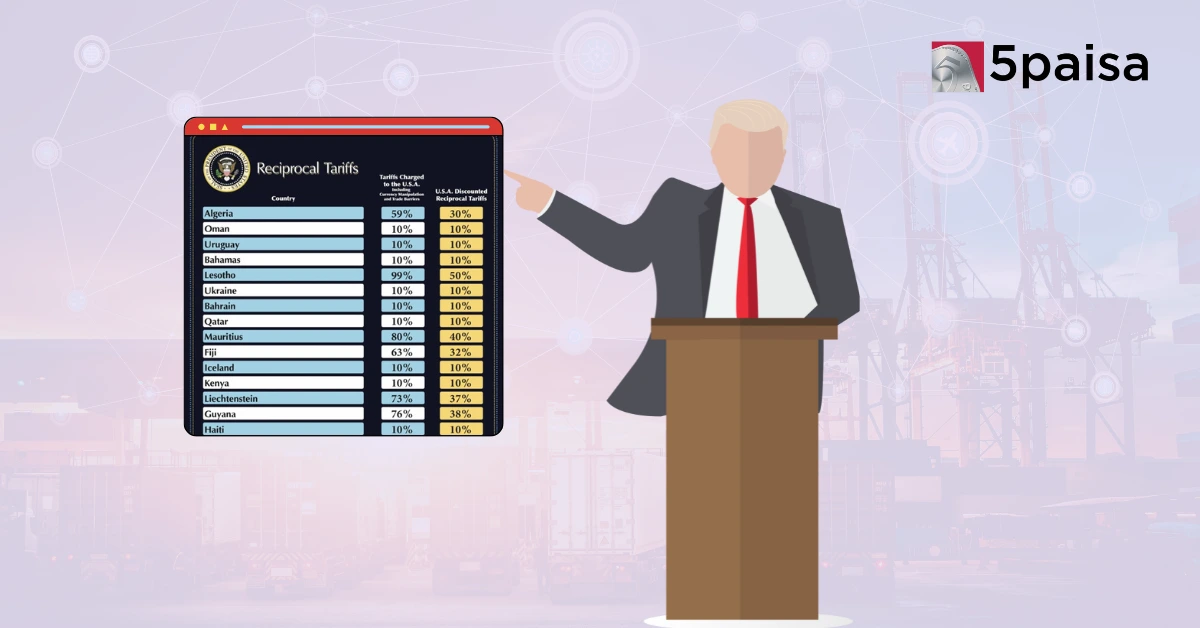
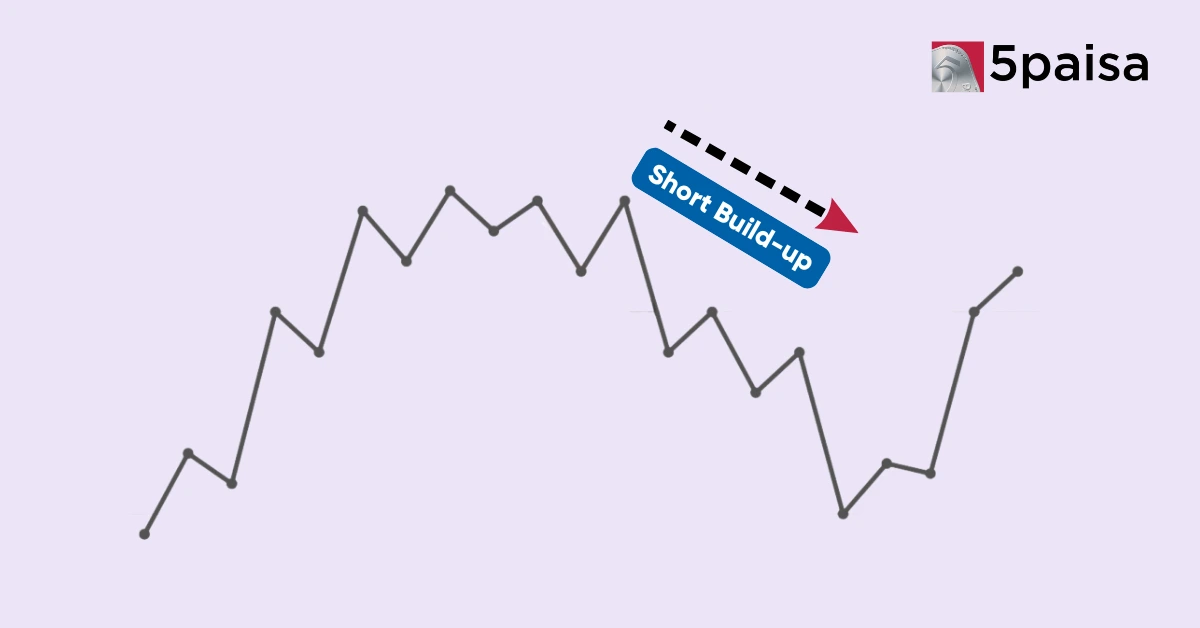
Iron Condor with Weekly Expiries: Is It Worth the Risk?
What is Continuous Compounding?

Introduction
Continuous compounding is needed to calculate the rates of interest, which are crucial to running an economy. People look for the interest percentage before opening an FD account or investing in any portfolio, as the rate of interest is calculated using different means. One of the ways of calculating the interest is through continuous compounding. In this article, let’s understand what is continuous compounding and how you can calculate it.
What is Continuous Compounding?
Continuous compounding is the limit of compound interest calculation where the interest is reinvested into an account’s balance an infinite number of times. It increases the interest component and the value of the portfolio of the entire investment. Although it may not be practical in the real world, it is essential in the financial world.
Additionally, it is a sporadic compounding case since most of the accrued interest is compounded monthly, quarterly, semi-annually, or annually. In simple words, continuous compounding assumes that interest is compounded and added back to the source account an infinite number of times. It means that an account is regularly earning interest, reinvesting the same interest back in the balance, and again earning interest on it.
Importance of Continuous Compounding
Here are some of the reasons why continuous compounding is essential:
1. It shows how much balance can be earned when the interest is accruing.
2. It helps investors calculate how much they can expect from their investment, earning a continuously compounding interest.
3. It further helps the investors to make a sound decision on where to reinvest this earned interest to make more profit.
4. It also increases the amount faster than simple interest, as the latter is only calculated on the principal amount.
5. Through compounding, money is multiplied at an increased rate. The greater the compounding periods, the more compound interest will be.
6. It can also boost investment returns, specifically in the long run.
Continuous Compounding Formula
Now that you have understood the continuous compounding meaning, it is time to look at its formula. Below is the compounded continuously formula:
A= Pert
Calculation of Compounding Formula
Here in this section, let’s understand the different elements of the continuous compound interest formula:
A= Pert
In the above,
A = The final amount
P = The initial amount
R = The interest rate
T = Time
E = the mathematical constant, where e = 2.7183
In this calculation, the time changes on how it is planned to be compounded. If it is quarterly, the time will be 1/4th.
If it is done bi-annually, the time will be 1/2th; if it is done annually, it would be 1/365.
The calculation can even happen on an hourly, minute, or daily basis as well. And in practical terms, that makes no value since the difference will only be in decimal points.
How To Derive Continuous Compounding Formula?
The continuous compounding formula is derived from the compound interest formula. The compound interest formula is as below:
A = P (1 + r/n)nt
In the above,
A = the final amount or can also be called the future value
P = the initial amount
N = it is the number of times P or the initial amount is compounding
T = time
R = rate of interest
In the case of the continuous compound interest, n → ∞. So, the limit in the above formula is
A = limn→∞ P (1 + r/n)nt = Pert
Later on, in the final step, one of the limit formulas is used: limn→∞ (1 + r/n)n = er.
And from there, the continuous compounding formula is derived, which is
A= Pert
Examples Of How To Use Continuous Compounding
Let’s understand using the formula with the help of an example.
For example: The initial amount deposited in the bank is Rs. 2340 on a rate of interest: 3.1% on an annual basis. What will be the balance after three (3) years?
So, the formula says, A= Pert
From the above example, P = 2340,
r = 3.1, which will be 3.1/100 = 0.031
t = 3 (since we need to calculate for 3 years)
e = Napier's number, which is approximately 2.7183
Let’s calculate:
A = 2340 e0.031(3) ≈ 2568.06
So, after three years, the amount received will be Rs. 2568.05
Simple Interest v/s Compound Interest
There is a difference in how both these are calculated.
Simple interest is the interest received on the initial principal amount after a fixed term. In such cases, the interest is not added to the initial amount. The interest is paid on the initial amount at a specified time.
While on the other hand, in Compound interest, the initial principal amount changes to accommodate the earned interest. So every year, the amount you will receive will have the previous year's interest added to the initial principal amount.
Conclusion
The formula checks the earned interest on the initial principal amount. In this case, the compounding time is often considered as infinity. Although continuous compounding shows the final value of the amount to be higher than the simple interest, it is challenging to apply in real-world cases. Therefore, it may not be too practical to use in real life as it holds significant importance in the financial world.
Instead, it holds value from the investor's end. Investors can check the amount they will receive on an ‘X’ investment and decide on future investment plans.
- Flat ₹20 Brokerage
- Next-gen Trading
- Advance Charting
- Actionable Ideas
Trending on 5paisa
03
 5paisa Research Team
5paisa Research Team
Indian Stock Market Related Articles
Disclaimer: Investment in securities market are subject to market risks, read all the related documents carefully before investing. For detailed disclaimer please Click here.

 5paisa Research Team
5paisa Research Team